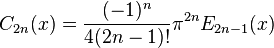Bernoulli Polynomials - Fourier Series

Fourier Series

The Fourier series of the Bernoulli polynomials is also a Dirichlet series, given by the expansion

Note the simple large n limit to suitably scaled trigonometric functions.

This is a special case of the analogous form for the Hurwitz zeta function

B_n(x) = -\Gamma(n+1) \sum_{k=1}^\infty \frac{ \exp (2\pi ikx) + e^{i\pi n} \exp (2\pi ik(1-x)) } { (2\pi ik)^n }.

This expansion is valid only for 0 ≤ x ≤ 1 when n ≥ 2 and is valid for 0 < x < 1 when n = 1.

The Fourier series of the Euler polynomials may also be calculated. Defining the functions

C_\nu(x) = \sum_{k=0}^\infty \frac {\cos((2k+1)\pi x)} {(2k+1)^\nu}

and

S_\nu(x) = \sum_{k=0}^\infty \frac {\sin((2k+1)\pi x)} {(2k+1)^\nu}

for, the Euler polynomial has the Fourier series

C_{2n}(x) = \frac{(-1)^n}{4(2n-1)!} \pi^{2n} E_{2n-1} (x)

and

S_{2n+1}(x) = \frac{(-1)^n}{4(2n)!} \pi^{2n+1} E_{2n} (x).

Note that the and are odd and even, respectively:

and

They are related to the Legendre chi function as

and

Read more about this topic:  Bernoulli Polynomials

Famous quotes containing the word series:

    “The theory of truth is a series of truisms.”
    —J.L. (John Langshaw)