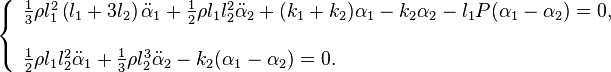Buckling - Flutter Instability

Flutter Instability

Structures subject to a follower (nonconservative) load may suffer instabilities which are not of the buckling type and therefore are not detectable with a static approach. For instance, the so-called 'Ziegler column' is shown in Fig.5.

This two-degree-of-freedom system does not display a quasi-static buckling, but becomes dynamically unstable. To see this, we note that the equations of motion are

\ \left\{ \begin{array}{l} \frac{1}{3} \rho l_1^{2} \left(l_1 + 3 l_2\right)\ddot{\alpha}_1 + \frac{1}{2} \rho l_1 l_2^{2} \cos(\alpha_1 - \alpha_2)\ddot{\alpha}_2 + \frac{1}{2} \rho l_1 l_2^{2} \sin(\alpha_1 - \alpha_2)\dot{\alpha}_2^{2} + (k_1 + k_2)\alpha_1 - k_2\alpha_2\,+ \\ + (\beta_1 + \beta_2)\dot{\alpha}_1 - \beta_2 \dot{\alpha}_2 - l_1 P \sin(\alpha_1 - \alpha_2) = 0, \\ \frac{1}{2} \rho l_1 l_2^{2} \cos(\alpha_1 - \alpha_2)\ddot{\alpha}_1 + \frac{1}{3} \rho l_2^{3}\ddot{\alpha}_2 - \frac{1}{2} \rho l_1 l_2^{2} \sin(\alpha_1 - \alpha_2)\dot{\alpha}_1^{2} - k_2(\alpha_1 - \alpha_2) - \beta_2(\dot{\alpha}_1 - \dot{\alpha}_2) = 0 , \end{array} \right.

and their linearized version is

\ \left\{ \begin{array}{l} \frac{1}{3} \rho l_1^{2} \left(l_1 + 3 l_2\right)\ddot{\alpha}_1 + \frac{1}{2} \rho l_1 l_2^{2} \ddot{\alpha}_2 + (k_1 + k_2)\alpha_1 - k_2\alpha_2 - l_1 P (\alpha_1 - \alpha_2) = 0, \\ \frac{1}{2} \rho l_1 l_2^{2} \ddot{\alpha}_1 + \frac{1}{3} \rho l_2^{3}\ddot{\alpha}_2 - k_2(\alpha_1 - \alpha_2) = 0 . \end{array} \right.

Assuming a time-harmonic solution in the form

we find the critical loads for flutter and divergence ,

where and .

Flutter instability corresponds to a vibrational motion of increasing amplitude and is shown in Fig.6 (upper part) together with the divergence instability (lower part) consisting in an exponential growth.

Recently, Bigoni and Noselli (2011) have experimentally shown that flutter and divergence instabilities can be directly related to dry friction, watch the movie for more details.

Read more about this topic:  Buckling

Famous quotes containing the words flutter and/or instability:

    “After the brief bivouac of Sunday,
    their eyes, in the forced march of Monday to Saturday,
    hoist the white flag, flutter in the snow storm of paper,”
    —Patricia K. Page (b. 1916)

    “Not only does the wind of accidents stir me according to its blowing, but I am also stirred and troubled by the instability of my attitude.”
    —Michel de Montaigne (1533–1592)