Dice - Probability

Probability

For a single roll of a fair s-sided die, the probability of rolling each value is exactly 1/s; this is an example of a discrete uniform distribution. For n multiple rolls, with a s-sided die the possibility space is equal to sn. So, for n rolls of an s-sided die the probability of any result is 1/sn.

However, if we are rolling two dice and adding the result together, as in the game craps, the total is distributed in a triangular curve; the case for common dice follows:

Sum 2 3 4 5 6 7 8 9 10 11 12
Probability 1/36
 2/36
=1/18		 3/36
=1/12		 4/36
=1/9		 5/36
 6/36
=1/6		 5/36
 4/36
=1/9		 3/36
=1/12		 2/36
=1/18		 1/36

As the number of dice increases, the distribution of the sum of all numbers tends to normal distribution by the central limit theorem; the exact value of a sum of n s-sided dice, k, is

where Fs,1(k) = 1/s for 1 ≤ k ≤ s and 0 otherwise.

A faster algorithm would adapt the exponentiation by squaring algorithm:

.

In the triangular curve described above,

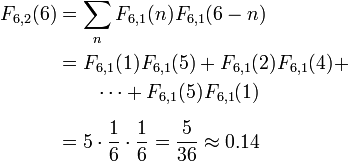
\begin{align} F_{6,2}(6) & =\sum_n {F_{6,1}(n) F_{6,1}(6 - n)} \\ & =F_{6,1}(1) F_{6,1}(5) + F_{6,1}(2) F_{6,1}(4) + \\ & \qquad \cdots + F_{6,1}(5) F_{6,1}(1) \\ & = 5\cdot\frac{1}{6}\cdot\frac{1}{6}=\frac{5}{36}\approx0.14 \end{align}

Equivalently, the probability can be calculated using combinations:

where is the floor function. The probability of rolling an exact sequence of numbers is 1/sn.

Read more about this topic:  Dice

Famous quotes containing the word probability:

    “Crushed to earth and rising again is an author’s gymnastic. Once he fails to struggle to his feet and grab his pen, he will contemplate a fact he should never permit himself to face: that in all probability books have been written, are being written, will be written, better than anything he has done, is doing, or will do.”
    —Fannie Hurst (1889–1968)

    “The source of Pyrrhonism comes from failing to distinguish between a demonstration, a proof and a probability. A demonstration supposes that the contradictory idea is impossible; a proof of fact is where all the reasons lead to belief, without there being any pretext for doubt; a probability is where the reasons for belief are stronger than those for doubting.”
    —Andrew Michael Ramsay (1686–1743)

    “Liberty is a blessing so inestimable, that, wherever there appears any probability of recovering it, a nation may willingly run many hazards, and ought not even to repine at the greatest effusion of blood or dissipation of treasure.”
    —David Hume (1711–1776)