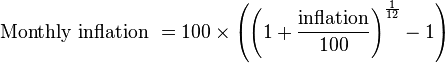Units of Inflation
Inflation rate is usually measured in percent per year. It can also be measured in percent per month or in price doubling time.
Example of inflation rates and units
When first bought, an item cost 1 currency unit. Later, the price rose...
| Old price |
New price 1 year later |
New price 10 years later |
New price 100 years later |
(Annual) inflation |
Monthly
inflation
|
Price
doubling
time
|
Zero add time |
| 1 |
|
|
|
0.01 |
|
|
23028 |
| 1 |
|
|
|
0.1 |
|
|
2300 |
| 1 |
|
|
|
0.3 |
|
|
769 |
| 1 |
|
|
|
1 |
|
|
231 |
| 1 |
|
|
|
3 |
|
|
77.9 |
| 1 |
|
|
|
10 |
|
|
24.1 |
| 1 |
|
|
|
100 |
|
|
3.32 |
| 1 |
|
|
|
900 |
|
|
1 |
| 1 |
|
|
|
3000 |
|
|
0.671 (8 months) |
| 1 |
|
|
|
1014 |
|
|
0.0833 (1 month) |
| 1 |
|
|
|
1.67 × 1075 |
|
|
0.0137 (5 days) |
| 1 |
|
|
|
1.05 × 102,639 |
|
|
0.000379 (3.3 hours) |

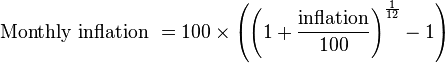


Often, at redenominations, three zeroes are cut from the bills. It can be read from the table that if the (annual) inflation is for example 100%, it takes 3.32 years to produce one more zero on the price tags, or 3 × 3.32 = 9.96 years to produce three zeroes. Thus can one expect a redenomination to take place about 9.96 years after the currency was introduced.