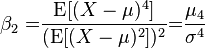Pearson Moments
The fourth standardized moment is defined as
where μ4 is the fourth moment about the mean and σ is the standard deviation. This is sometimes used as the definition of kurtosis in older works, but is not the definition used here.
Kurtosis is more commonly defined as the fourth cumulant divided by the square of the second cumulant, which is equal to the fourth moment around the mean divided by the square of the variance of the probability distribution minus 3,
which is also known as excess kurtosis. The "minus 3" at the end of this formula is often explained as a correction to make the kurtosis of the normal distribution equal to zero. Another reason can be seen by looking at the formula for the kurtosis of the sum of random variables. Suppose that Y is the sum of n identically distributed independent random variables all with the same distribution as X. Then
This formula would be much more complicated if kurtosis were defined just as μ4 / σ4 (without the minus 3).
More generally, if X1, ..., Xn are independent random variables, not necessarily identically distributed, but all having the same variance, then
whereas this identity would not hold if the definition did not include the subtraction of 3.
The fourth standardized moment must be at least 1, so the excess kurtosis must be −2 or more. This lower bound is realized by the Bernoulli distribution with p = ½, or "coin toss". There is no upper limit to the excess kurtosis and it may be infinite.
Read more about this topic: Kurtosis
Famous quotes containing the words pearson and/or moments:
“Education is considered the peculiar business of women; perhaps for that very reason it is one of the worst-paid businesses in the world ...”
—Katharine Pearson Woods (1853–1923)
“The essence of the modern state is that the universal be bound up with the complete freedom of its particular members and with private well-being, that thus the interests of family and civil society must concentrate themselves on the state.... It is only when both these moments subsist in their strength that the state can be regarded as articulated and genuinely organized.”
—Georg Wilhelm Friedrich Hegel (1770–1831)