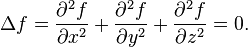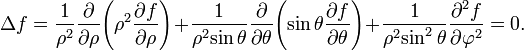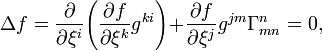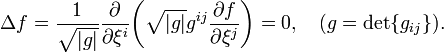Laplace's Equation - Definition

Definition

In three dimensions, the problem is to find twice-differentiable real-valued functions, of real variables x, y, and z, such that

In Cartesian coordinates

\Delta f = {\partial^2 f\over \partial x^2 } + {\partial^2 f\over \partial y^2 } + {\partial^2 f\over \partial z^2 } = 0.

In cylindrical coordinates,

\Delta f = {1 \over r} {\partial \over \partial r} \left( r {\partial f \over \partial r} \right) + {1 \over r^2} {\partial^2 f \over \partial \phi^2} + {\partial^2 f \over \partial z^2 } =0

In spherical coordinates,

\Delta f = {1 \over \rho^2}{\partial \over \partial \rho}\!\left(\rho^2 {\partial f \over \partial \rho}\right) \!+\!{1 \over \rho^2\!\sin\theta}{\partial \over \partial \theta}\!\left(\sin\theta {\partial f \over \partial \theta}\right) \!+\!{1 \over \rho^2\!\sin^2\theta}{\partial^2 f \over \partial \varphi^2} =0.

In Curvilinear coordinates,

\Delta f = {\partial \over \partial \xi^i}\!\left({\partial f \over \partial \xi^k}g^{ki}\right) \!+\!{\partial f \over \partial \xi^j}g^{jm}\Gamma^n_{mn} =0,

or

\Delta f = {1 \over \sqrt{|g|}}{\partial \over \partial \xi^i}\!\left(\sqrt{|g|}g^{ij}{\partial f \over \partial \xi^j}\right) =0, \quad (g=\mathrm{det}\{g_{ij}\}).

This is often written as

or, especially in more general contexts,

where ∆ = ∇² is the Laplace operator or "Laplacian"

where ∇ ⋅ = div is the divergence, and ∇ = grad is the gradient.

If the right-hand side is specified as a given function, f(x, y, z), i.e., if the whole equation is written as

then it is called "Poisson's equation".

The Laplace equation is also a special case of the Helmholtz equation.

Read more about this topic:  Laplace's Equation

Famous quotes containing the word definition:

    “The man who knows governments most completely is he who troubles himself least about a definition which shall give their essence. Enjoying an intimate acquaintance with all their particularities in turn, he would naturally regard an abstract conception in which these were unified as a thing more misleading than enlightening.”
    —William James (1842–1910)

    “Beauty, like all other qualities presented to human experience, is relative; and the definition of it becomes unmeaning and useless in proportion to its abstractness. To define beauty not in the most abstract, but in the most concrete terms possible, not to find a universal formula for it, but the formula which expresses most adequately this or that special manifestation of it, is the aim of the true student of aesthetics.”
    —Walter Pater (1839–1894)

    “The definition of good prose is proper words in their proper places; of good verse, the most proper words in their proper places. The propriety is in either case relative. The words in prose ought to express the intended meaning, and no more; if they attract attention to themselves, it is, in general, a fault.”
    —Samuel Taylor Coleridge (1772–1834)