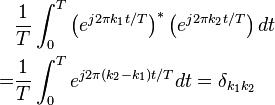Orthogonal Frequency-division Multiplexing - Mathematical Description

Mathematical Description

If sub-carriers are used, and each sub-carrier is modulated using alternative symbols, the OFDM symbol alphabet consists of combined symbols.

The low-pass equivalent OFDM signal is expressed as:

\ \nu(t)=\sum_{k=0}^{N-1}X_k e^{j2\pi kt/T}, \quad 0\le t<T,

where are the data symbols, is the number of sub-carriers, and is the OFDM symbol time. The sub-carrier spacing of makes them orthogonal over each symbol period; this property is expressed as:

\begin{align} &\frac{1}{T}\int_0^{T}\left(e^{j2\pi k_1t/T}\right)^* \left(e^{j2\pi k_2t/T}\right)dt \\ = &\frac{1}{T}\int_0^{T}e^{j2\pi (k_2-k_1)t/T}dt = \delta_{k_1k_2} \end{align}

where denotes the complex conjugate operator and is the Kronecker delta.

To avoid intersymbol interference in multipath fading channels, a guard interval of length is inserted prior to the OFDM block. During this interval, a cyclic prefix is transmitted such that the signal in the interval equals the signal in the interval . The OFDM signal with cyclic prefix is thus:

The low-pass signal above can be either real or complex-valued. Real-valued low-pass equivalent signals are typically transmitted at baseband—wireline applications such as DSL use this approach. For wireless applications, the low-pass signal is typically complex-valued; in which case, the transmitted signal is up-converted to a carrier frequency . In general, the transmitted signal can be represented as:

\begin{align} s(t) & = \Re\left\{\nu(t) e^{j2\pi f_c t}\right\} \\ & = \sum_{k=0}^{N-1}|X_k|\cos\left(2\pi t + \arg\right) \end{align}

Read more about this topic:  Orthogonal Frequency-division Multiplexing

Famous quotes containing the words mathematical and/or description:

    “It is by a mathematical point only that we are wise, as the sailor or the fugitive slave keeps the polestar in his eye; but that is sufficient guidance for all our life. We may not arrive at our port within a calculable period, but we would preserve the true course.”
    —Henry David Thoreau (1817–1862)

    “The Sage of Toronto ... spent several decades marveling at the numerous freedoms created by a “global village” instantly and effortlessly accessible to all. Villages, unlike towns, have always been ruled by conformism, isolation, petty surveillance, boredom and repetitive malicious gossip about the same families. Which is a precise enough description of the global spectacle’s present vulgarity.”
    —Guy Debord (b. 1931)