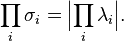QR Decomposition - Connection To A Determinant or A Product of Eigenvalues

Connection To A Determinant or A Product of Eigenvalues

We can use QR decomposition to find the absolute value of the determinant of a square matrix. Suppose a matrix is decomposed as . Then we have

Since Q is unitary, . Thus,

where are the entries on the diagonal of R.

Furthermore, because the determinant equals the product of the eigenvalues, we have

where are eigenvalues of .

We can extend the above properties to non-square complex matrix by introducing the definition of QR-decomposition for non-square complex matrix and replacing eigenvalues with singular values.

Suppose a QR decomposition for a non-square matrix A:

where is a zero matrix and is an unitary matrix.

From the properties of SVD and determinant of matrix, we have

where are singular values of .

Note that the singular values of and are identical, although the complex eigenvalues of them may be different. However, if A is square, it holds that

{\prod_{i} \sigma_{i}} = \Big|{\prod_{i} \lambda_{i}}\Big|.

In conclusion, QR decomposition can be used efficiently to calculate a product of eigenvalues or singular values of matrix.

Read more about this topic:  QR Decomposition

Famous quotes containing the words connection to, connection and/or product:

    “It may comfort you to know that if your child reaches the age of eleven or twelve and you have a good bond or relationship, no matter how dramatic adolescence becomes, you children will probably turn out all right and want some form of connection to you in adulthood.”
    —Charlotte Davis Kasl (20th century)

    “The Transcendentalist adopts the whole connection of spiritual doctrine. He believes in miracle, in the perpetual openness of the human mind to new influx of light and power; he believes in inspiration, and in ecstacy.”
    —Ralph Waldo Emerson (1803–1882)

    “Culture is a sham if it is only a sort of Gothic front put on an iron building—like Tower Bridge—or a classical front put on a steel frame—like the Daily Telegraph building in Fleet Street. Culture, if it is to be a real thing and a holy thing, must be the product of what we actually do for a living—not something added, like sugar on a pill.”
    —Eric Gill (1882–1940)