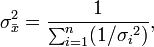Weighted Mean - Dealing With Variance

Dealing With Variance

See also: Least squares#Weighted least squares See also: Linear least squares (mathematics)#Weighted linear least squares

For the weighted mean of a list of data for which each element comes from a different probability distribution with known variance, one possible choice for the weights is given by:

w_i = \frac{1}{\sigma_i^2}.

The weighted mean in this case is:

\bar{x} = \frac{ \sum_{i=1}^n (x_i/{\sigma_i}^2)}{\sum_{i=1}^n (1/{\sigma_i}^2)},

and the variance of the weighted mean is:

\sigma_{\bar{x}}^2 = \frac{ 1 }{\sum_{i=1}^n (1/{\sigma_i}^2)},

which reduces to, when all

The significance of this choice is that this weighted mean is the maximum likelihood estimator of the mean of the probability distributions under the assumption that they are independent and normally distributed with the same mean.

Read more about this topic:  Weighted Mean

Famous quotes containing the words dealing with, dealing and/or variance:

    “The economic dependence of woman and her apparently indestructible illusion that marriage will release her from loneliness and work and worry are potent factors in immunizing her from common sense in dealing with men at work.”
    —Mary Barnett Gilson (1877–?)

    “The wisest thing a parent can do is to let preschool children figure out themselves how to draw the human figure, or solve a whole range of problems, from overcoming Saturday-morning boredom to dealing with a neighborhood bully. But even while standing on the sidelines, parents can frequently offer support in helping children discover what they want to accomplish.”
    —John F. Clabby (20th century)

    “There is an untroubled harmony in everything, a full consonance in nature; only in our illusory freedom do we feel at variance with it.”
    —Fyodor Tyutchev (1803–1873)